Without numbers, our lives would be difficult to imagine. Numbers are the building blocks of mathematics. People may believe that numbers are simply combinations of one to nine digits, however, this is not the case. Numbers have a wide range of qualities. We can distinguish them depending on any feature that they exhibit. If a number is divisible by two, for example, it belongs to the category of even numbers. Similarly, there is a great deal of number classification. In this article, we’ll look at two different types of number classifications. The subjects we will be discussing include irrational and rational numbers. They are extremely important in a variety of fields. As a result, students must have a thorough understanding of these issues.
Rational numbers
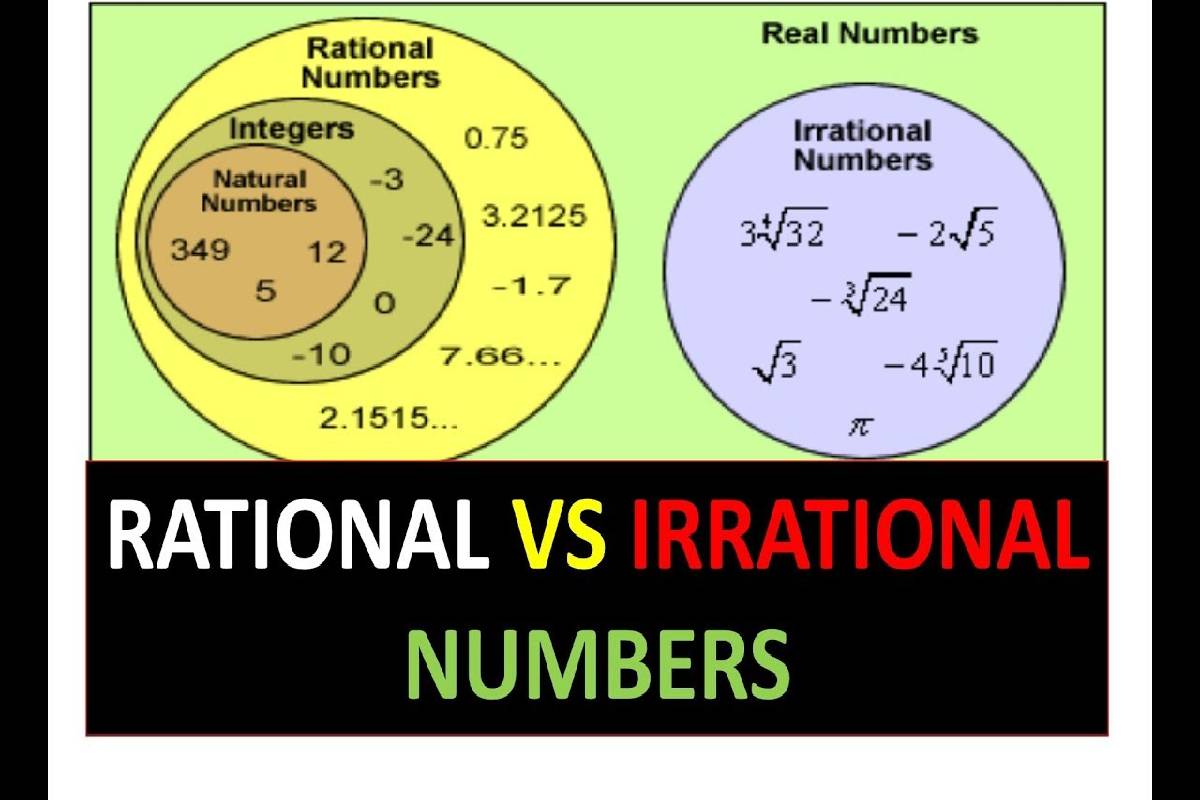
Rational numbers are numbers that are observed by everyone, not just students. If one will look at the rational word closely, he or she will notice that it has something to do with ratio. A rational number is one that can be expressed in the form p/q, where both p and q are integers and q is never equal to zero. Although it is likely that a person will become confused between rational numbers and fractions, they should be aware of the fundamental differences between the two. As previously stated, rational numbers are made up of integers, whereas fractions are made up of whole numbers.
Someone who believes that a rational number can only be of one type is mistaken. There are many different sorts of rational numbers. If an essential condition is met, decimal numbers can be considered rational numbers as well. The terminating decimal is also a rational number. The numbers that have an end are known as terminating decimals and never-ending decimals are termed as non-terminating decimals. It’s worth noting that all integers belong to the category of rational numbers. Because all integers are rational numbers, we can conclude that all whole numbers are rational as well. To solve problems involving numbers, the student should be familiar with rational numbers.
Irrational numbers
These are the numbers that can’t be expressed in terms of ratios. Irrational numbers are defined as numbers that are not rational numbers. A fraction can never be used to represent these quantities. Observing the decimal number is one approach to determine whether a number is irrational or not. When certain operations are performed on two irrational integers, the result can be either rational or irrational. The sum of irrational numbers, for example, can yield a rational or irrational number as an answer.
There are numerous examples of irrational numbers that we encounter on a daily basis. Pi is one of the most well-known irrational numbers, although square roots of 2, 3,5, and many other numbers are also irrational. Irrational numbers have no finite values, which no one can determine. Pi, for example, has an infinite value. Similarly, there are more such examples that help us to understand irrational numbers. On a regular basis, students must deal with irrational numbers. As a result, kids should be able to tell the difference between rational and irrational numbers.
We attempted to focus and cover both the issues of rational and irrational numbers in depth in the preceding post. Numbers are the most fundamental quantity that pupils must comprehend. Because if their fundamentals are clear, kids will have no trouble understanding higher-level mathematical issues. If students have trouble grasping concepts that are directly related to mathematics, they should seek help from Cuemath. It is an excellent platform that helps students in clearing their doubts related to Coding and Mathematics.